- Home
- General Aptitude
- Height and Distance
6.
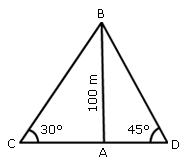
Two ships are sailing in the sea on the two sides of a lighthouse. The angles of elevation of the top of the lighthouse as observed from the two ships are 30° and 45° respectively. If the lighthouse is 100 m hight, the distance between the two ships is:
- A.173 m
- C.273 m
- B.200 m
- D.300 m
- Answer & Explanation
- Report
Answer : [C]
Explanation :
Explanation :
|
 |
7.
The top of a 15 metre high tower makes an angle of elevation of 60° with the bottom of an electronic pole and angle of elevation of 30° with the top of the pole. What is the height of the electric pole?
- A.5 metres
- C.10 metres
- E.None of these
- B.8 metres
- D.12 metres
- Answer & Explanation
- Report
Answer : [C]
Explanation :
Explanation :
|
 |
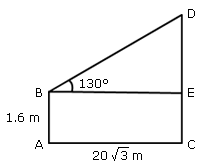
8.
|
|||
| the top of the tower is 30. The height of the tower is: |
- A.21.6 m
- C.24.72 m
- B.23.2 m
- D.None of these
- Answer & Explanation
- Report
Answer : [A]
Explanation :
Explanation :
|
 |
9.
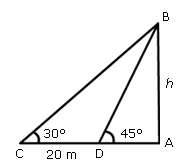
The angle of elevation of the top of a tower from a certain point is 30°. If the observer moves 20 m towards the tower, the angle of elevation of the top of the tower increases by 15°. The height of the tower is:
- A.17.3 m
- C.27.3 m
- B.21.9 m
- D.30 m
- Answer & Explanation
- Report
Answer : [C]
Explanation :
Explanation :
|
 |
10.
On the same side of a tower, two objects are located. Observed from the top of the tower, their angles of depression are 45° and 60°. If the height of the tower is 150 m, the distance between the objects is:
- A.63.5 m
- C.86.7 m
- B.76.9 m
- D.90 m
- Answer & Explanation
- Report
Answer : [A]
Explanation :
Explanation :
|
 |